Operations with Monomials
A monomial is an algebraic expression that is a constant or a
product of a constant and one or
more variables with whole number exponents.
Monomials:
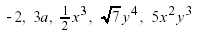
Basic Rules for Exponents
1. Extending the Multiplying of “powers with the same baseâ€: a m
· a n
· a p
=a m + n + p
Add exponents by the rules of adding signed numbers where m, n, p
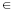 { integers} { integers}
Example 1:
a) z 5 · z 2
· z 4 = z 5+2+4 = z 11
b) x 2 · x 3
· x 4
· x 5 = x 2+3+4+5 = x
14
2. Powering of powers:
For base a and exponents m, n
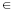 { natural numbers
} { natural numbers
}
(a m) n = a m·n
Example 2:
a) (x 4 )3 = x 4·3 = x 12
b) (y 2) 5 = y2·5 = y 10
3. Powering of products with different bases a and b, and exponent n
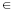 { natural numbers} { natural numbers}
(a·b) n = a
n · b n
NOTE: The exponent affects only the number that it
immediately touches so you must always put
negative numbers in parentheses ( ).
Example 3:
1. (x 5 y 7)3 = (x 5)3·(y
7)3 = x5·3·y7·3 = x15 y21 Apply properties and multiply exponents.
2. (-2 · x2y3)4 = (-2)4
·(x2)4·(y3)4 = 16
·(x2·4)·(y3·4) = 16
· x8y12 Apply properties, multiply exponents.
Definition of Negative Exponent
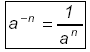 Note: a -n × a n = 1 They are reciprocals.
Note: a -n × a n = 1 They are reciprocals.
The sign in the exponent does not affect the sign of the number in the base.
Example 4:
a)
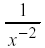 Since -2 is in the denominator place positive in the numerator: Since -2 is in the denominator place positive in the numerator:

b) x -8 Since -8 is in the numerator place positive in the denominator:
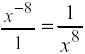
Definition of Zero Exponent a 0 = 1 Note: The result of raising any base to the 0 exponent
will always be 1. (a ≠ 0)
1. (57x9 y32 z −25 )0 = 1
4. Division of powers with positive exponents:
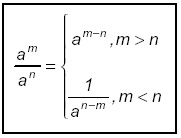 |
Subtract the smaller exponent from the larger
exponent and put the resulting “power †in the “placeâ€
of the larger “powerâ€.
Put a 1 in the numerator if the larger “power†is in the
denominator.
|
Example 5: (Working with positive exponents):
1.
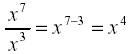 [7 > 3 result goes in the numerator.]
[7 > 3 result goes in the numerator.]
2.
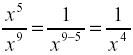 [9 > 5 result goes in the denominator.] [9 > 5 result goes in the denominator.]
3.
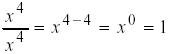 [4 = 4 Exponents the same result is always 1.]
[4 = 4 Exponents the same result is always 1.]
|